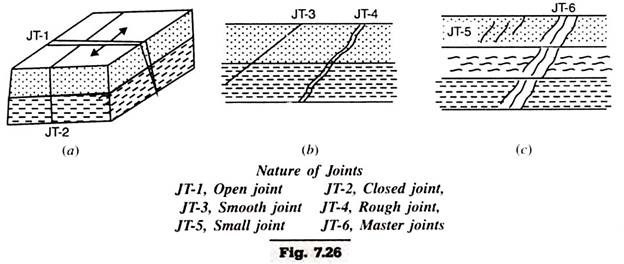
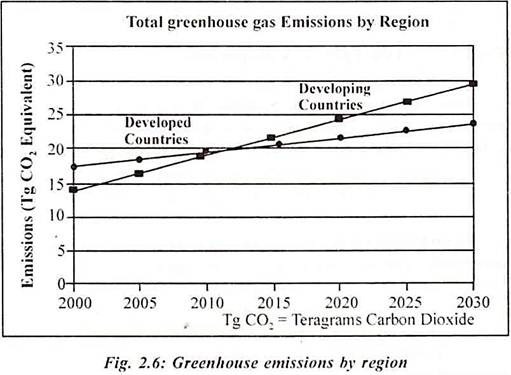
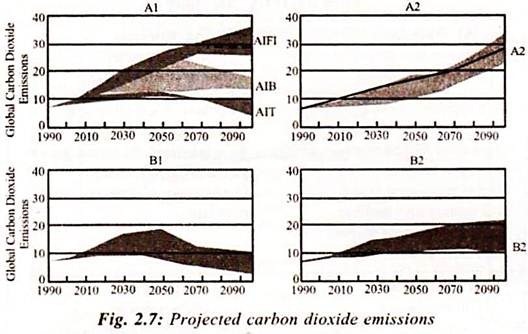
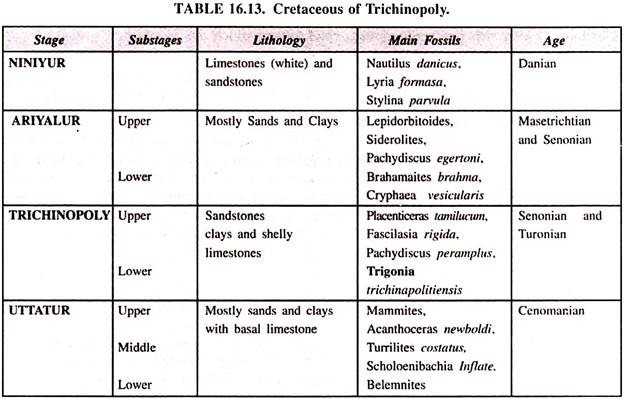
ADVERTISEMENTS:
In this article we will discuss about:- 1. Types of Aquifers 2. Coefficient of Storage of Aquifers 3. Dupuit’s Theory 4. Coefficient of Transmissibility 5. Confined Aquifers Well.
Contents:
- Types of Aquifers
- Coefficient of Storage of Aquifers
- Dupuit’s Theory for Aquifers
- Coefficient of Transmissibility of Aquifers
- Confined Aquifers Well
1. Types of Aquifers:
ADVERTISEMENTS:
Aquifers are mainly of two types:
1. Unconfined Aquifer:
The topmost water bearing strata having no confining impermeable over-burden is known as unconfined aquifer. This aquifer is also known as non-artesian aquifer or water table aquifer. Water table in such an aquifer varies in undulating form depending upon the storage of water within it. The gravity wells are constructed to tap water from unconfined aquifer only. The water rise in such wells is equal to water rise in a piezometer connected to the water table.
2. Confined Aquifer:
It is such an aquifer which remains overladen by an impermeable strata or aquiclude. The water is under pressure in this aquifer. Confined aquifers are also known as artesian aquifers. They can be considered analogous to pipe lines flowing under pressure. The static pressure at a point in a confined aquifer is equal to the elevation of the water table recharging the area minus the loss of head through the aquifer to the point under consideration.
ADVERTISEMENTS:
The rise of water in the well, taping confined aquifer is determined by connecting a piezometer tube to this layer and noting the rise of water in the tube. Artesian wells are those which are constructed sufficiently deep to tap water from 2nd or even lower water bearing strata. These wells are generally small in size with diameters less than 30 cm.
When pressure of water in a certain water bearing formation is so high that water rises above the ground level automatically such a well is known as flowing well. If, however, the water level in such a well remain below the ground level, but is above the local water table it is known as the artesian well.
The aquifers can be classified as confined or unconfined depending upon the absence or presence of water table within them. Confined aquifers do not have any Water Table as water in them is always under pressure. Unconfined aquifers are such formations which have equal pressure at all the points on its surface and pressure is equal to hydrostatic pressure. Therefore in an unconfined well, water will rise up to the water table level, but in confined well water will rise up to the piezometric head. The line joining the various pierometric heads in various tightly cased wells tapping the aquifer is known as piezometric surface.
ADVERTISEMENTS:
Perched Aquifer:
It is a special condition of aquifer which may exist in unconfined aquifer. If an impervious stratum of small area extent lying within zone of saturation is found to support a body of saturated soil above it, then this body of saturated soil is known as perched aquifer. The top surface of water held in the perched aquifer is known as perched water table.
2. Coefficient of Storage of Aquifers:
Water discharged from an aquifer or recharged into an aquifer represents the change in its storage volume. It is very easy to determine this change for unconfined aquifers. Rise or fall of water table in given time is determined experimentally. Multiplying this by average specific capacity during the time, the change of the storage volume can be found out.
In the case of confined aquifers, change in pressure produces only small change in storage volume. The hydrostatic pressure within an aquifer partially supports the over burden and partially the solid structure of the aquifer. When hydrostatic pressure is reduced by pumping water from a quifer, the load on it is increased and aquifer gets consequently compressed and thus forces some water out of it. In addition, lowering of the pressure causes expansion and subsequent release of water. The water yielding capacity of an artesian acquifer can be expressed by its storage coefficient, generally denoted by A.
The storage coefficient (A) for a confined aquifer is equal to the volume of the water released from the aquifer of unit cross-sectional area and full height, when piezometric surface depresses by unity. In general, storage co-efficient is defined as the volume of water than an aquifer releases or stores per unit surface area of the aquifer per unit change in the head normal to that surface.
3. Dupuit’s Theory for Aquifers:
The analysis of flow towards well through soil was first studied by Mr. Dupuit in 1863. The method of analysis as adopted by Mr. Dupuit was later modified by Mr. Thiem in 1906.
Flow or discharge formulae, for both the analysis, for confined and unconfined formations, have been derived as follows:
Level of water in the well which is not flowing is same as that of water table. When water is pumped from the well, its level is depressed and water from surrounding soil starts entering the well. As soon as water starts entering the well, a depression in water table surrounding the well is created.
This depression is called the draw dawn curve or the cone of depression. Draw down at any point away from well is the vertical distance by which a water table is lowered from the normal water table.
The value of R is selected by experience. The values of R may be taken varying from 100 to 300 m. Selection of R is done on approximate basis only, as discharge does not change by appreciable amount if R is changed within quite wide ranges.
Value of R can also be estimated by Sichardt expression given below:
where R and s are in metres and k is in m/sec.
If draw down observations are taken from two observation wells located at radial distances of r1 and r2 and if the depths of water in the wells are respectively h1 and h2, then expression (1) given above can be expressed as follows:
(Since H-h = s, H + h= s + 2h)
If h = L the length of the strainer then
Assumptions of Dupuit’s Theory:
Following are the some assumptions of Dupuit’s Theory:
1. Aquifer is homogeneous, isotropic and of infinite aerial extent.
2. The velocity of flow is r optional to tangent of hydraulic gradient and not sine.
3. The flow is horizontal and uniform throughout the vertical section.
4. Entire thickness of aquifer is contributing water to the well.
5. Coefficient of transmissibility remains constant at all places and all the time.
6. Flow is laminar and Darcy’s law is applicable.
7. Ground water conditions remain constant all the time.
4. Coefficient of Transmissibility of Aquifers:
(a) Confined Aquifers:
The value of T can be determined by conducting prolonged pumping test and observing draw down at various observation wells. But essential feature of this method is that pumping must be at a uniform rate for sufficiently long time so that steady state conditions may develop. Steady state condition is one at which draw down changes negligibly with time. Refer Fig. 7.8.
S1 = draw down in well 1 = H-h1
s2 = draw down in well 2 = H-h2
h2 – h1 = (H – s2) – (H – s1) = s1 – s2
Where Δs = difference in draw downs at the two wells so selected that
r2= 10 r1
In this method, draw down observations s1, s2, s3……… sx are taken in observation wells located at r1, r2, r3……….. rx distance from the centre of the main well. Now a graph is plotted by taking draw downs along ordinate and log10 rx as abscissa. The plot will be a straight line as shown in Fig. 7.9. From this plot the value of Δ s can be obtained for one log cycle of distance. After substituting this value in Eq. (12) value of T can be determined.
(b) Unconfined Aquifers:
Refer Fig. 7.7
If we compare Eqs. (12) and (14) we find them identical except that Δs’ in (14) is based on modified values of draw down whereas it is based on actual values of drawdown in case of Eq. (12).
In this case also a graph is drawn similar to one drawn in case of confined aquifer. But here values of draw downs taken along ordinate are modified values of drawdowns. See Fig. 7. 10.
5. Confined Aquifers Well:
A well fully penetrating the confined aquifer is shown in Fig. 7.8. Let AB line represent initial piezometric surface and CD in the final position of water level in the well after pumping. Consider two observation wells 1 and 2 at radial distances of r1 and r2 from the centre of the well. Let s1 and s2 be the draw downs at observation wells 1 and 2 and h1 and h2 the depths of water in them respectively. Let H be the total height from impermeable layer lying below the confined aquifer up to initial piezometric surface. Let b be the thickness of confined aquifer.
Consider a point P on a draw down curve and let xy be its coordinates by considering point O, the centre point of the well at bottom as the origin.
Apply Darcy’s law for flow passing a vertical plane through point P.
Integrating between the limits [R, r] for x and H, h for y we get
By further simplifying
While considering the two observation wells 1 and 2 at radial distances of r1 and r2 the discharge Eq. (6) takes the shape as follows:
If hx is the depth at any radial distance rx the discharge Eq. (6) can be written as –
Equating Eqs (5) and (9), we get
Equation (10) shows that head varies linearly with the logarithm of the distance regardless of the rate of discharge.